Counting Overview
1. Overview
Developing number awareness at a young age helps children learn to think. The benefits go far beyond learning basic computation skills because a child’s oral abilities, motor skills, and memory are also developed.
Research shows starting early is a guaranteed way of getting your child ahead of the curve.
Early knowledge of math not only predicts later success in math, but also predicts later reading achievement even better than early reading skills.
– Education Commission of the States
2. Counting Concepts
In our Counting Curriculum, the activities are carefully planned to teach key concepts in a particular order. Children learn all the basic counting skills before studying more complicated ideas, such as estimation.
More specifically, our curriculum covers five fundamental skills: One-to-One Correspondence, Number Constancy, Subitizing, Cardinality, and Number Sequencing.
Once your child learns all these skills, he/she practices estimating, thinking analytically, adding mentally, and more.
2.1 One-to-one Correspondence

One-to-one Correspondence refers to counting each object once and only once. This avoids counting the same object three or four times. Children who don’t develop this skill will think there are more objects in front of them than are actually present.
2.2 Number Constancy
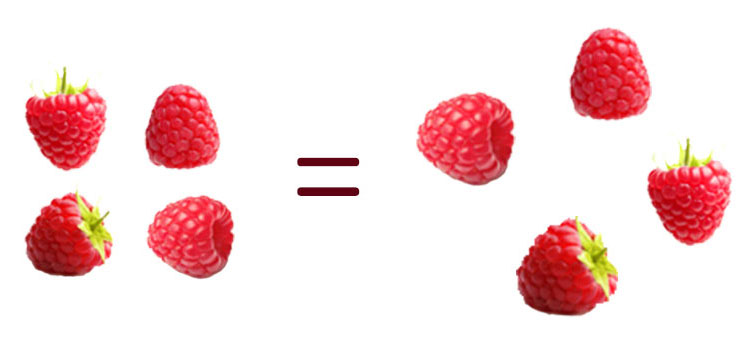
Number constancy is the idea that re-arranging or scattering a group of items doesn’t change the total number of objects in the group.
2.3 Subitizing
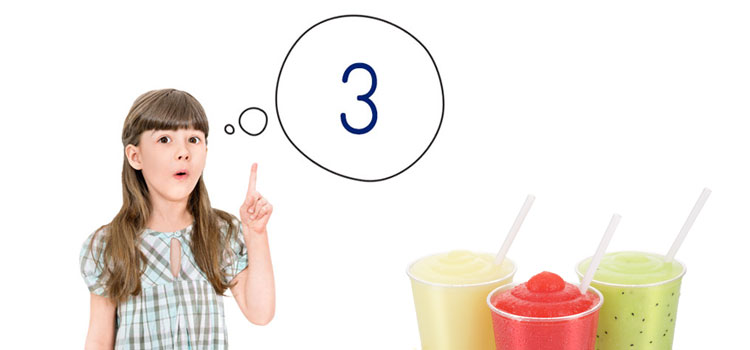
Subitizing refers to the ability to instantly count a group of objects. For example, being able to immediately count the number of dots on dice shows subitizing ability. This is important for advanced grouping.
2.4 Cardinality

Cardinality is the idea that the last number you counted is the same as the number of objects in a group. Cardinality is important for connecting a number with a physical group of objects and then making sense of that knowledge.
2.5 Number Sequencing
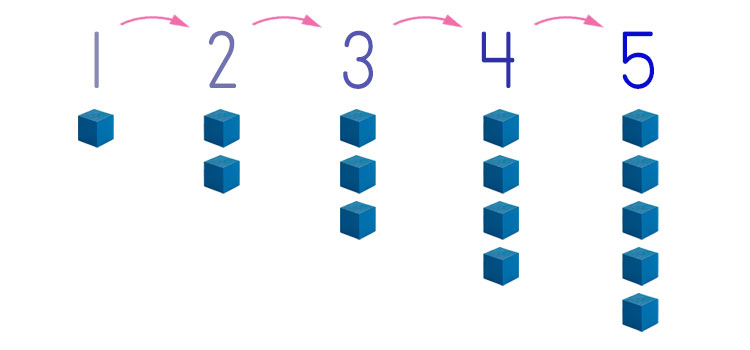
Number sequencing refers to understanding the sequential order of numbers. This is essential for addition, subtraction, and functionally any basic application of math.
3. Getting Started
The Sight Words Math program works best with children ages 3 through 5. It’s intended for bridging the gap between early childhood and kindergarten. The curriculum has around 40 games designed to teach children each of the five fundamental math concepts (outlined above) and a few more advanced concepts.
To get the most out of the curriculum, follow a pattern of repetition where you play two different games (one old and one new) every day. Establish a habit where you can set aside at least 15-20 minutes a day to play math games with your child. Continued practice is the best way to prepare your child for excellence.
For more details see the Curriculum page.
4. Research
Teaching children math at an early age establishes a solid foundation for critical thinking, mental calculation, and problem solving. By progressing in small steps, children build and reinforce skills necessary for future success in school, jobs, and daily life activities.
A focus on active learning, with several hands-on activities, encourages students to learn by doing. Children develop analytical skills as they react to problems and create their own solutions. Since a child’s early years spell out the course of their future psychological development, tapping their potential early is a guaranteed way to help your child succeed.
Here are a few examples of the research that has been done on this subject:
- D.H. Clements and J. Sarama. “Early Childhood Mathematics Education Research: Learning Trajectories for Young Children.” New York, NY: Routledge, 2009
- J. Sarama, A. Lange, D.H. Clements, and C.B. Wolfe, “The Impacts of an Early Mathematics Curriculum on Emerging Literacy and Language,” Early Childhood Research Quarterly, 27, 489-502, 2012, doi: 10.1016/j.ecresq.2011.12.002.
- National Mathematics Advisory Panel, “Foundations for Success: The Final Report of the National Mathematics Advisory Panel” (Washington D.C.: National Research Council, 2008)
- B. Wright, “What Number Knowledge Is Possessed by Children Beginning the Kindergarten Year of School?” Mathematics Education Research Journal, 3(1), 1991, 1-16.
- 0 B. Doig, B. McCrae, and K. Rowe, “A Good Start to Numeracy: Effective Numeracy Strategies from Research and Practice in Early Childhood” (Canberra ACT, Australia, 2003)
- National Research Council, “Mathematics in Early Childhood: Learning Paths toward Excellence and Equity” (Washington, DC: National Academy Press, 2009).
Leave a Reply

One Response to “Counting Overview”
Gail Stobie
I was wondering if the 2.1 cards and the others are available as a download. I have looked through the math curriculum and could not find them.
Also, your sight and materials are fantastic. They along with the videos are an amazing resource.
Thanks.
Gail